Squares on the sides of Parallelograms
By Leighton McIntyre
Goal : To investigate squares on the sides of a parallelogram
1)
Squares on the outside of Parallelogram
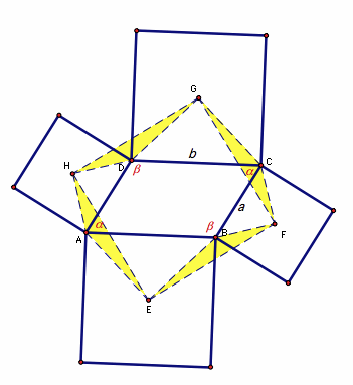
Given parallelogram ABCD with squares on the sides. Let a be the short side of the parallelogram.
Thus the sides of the two small squares will be a. Let b be the long side
of the parallelogram. Thus the sides of the two larger squares will be b. denote by a and b the
smaller and larger angles of the parallelogram.
The midpoint F of one of the smaller triangles on Side BC
of the parallelogram. The distance FB = FC = 
= HD = HA in the other small square, by corresponding
segments of congruent squares
The midpoint E of one of the larger triangles on Side AB
of the parallelogram. The distance EB = EA = 
. .= GD = GC in the other large square, by corresponding
segments of congruent squares. Consider <BFC in the small triangle is right,
because it is the angle formed by the intersection diagonals of a square.
Analogously <AEB is right angle.
The triangles formed by the sides of the parallelogram and
the midpoints of the squares are isosceles triangles. Consequently the angles
formed by the vertices of the parallelogram and the midpoint of the square are
450 each.
Consider <FCG = a + 90
Consider <FBE = 360 – (b + 90) = 360 – (180- a + 90) =
180 + a - 90 = a + 90
D FCG 
D FBE by
SAS congruence, particularly FGÈ FE.
Consequently, the quadrilateral FEHG has four congruent sides.
Next try to show that one of the angles in FEHG is right
angle.
Consider <HEF.
We know <AEB is right angle and <AEHÈ < BEF
<AEB = <AEH + <HEB = 90 = <HEB + <BEF =
<HEF
Thus quadrilateral FEHG is a square,
2) What
if the squares are on the inside of the parallelogram?
Let small angle and larger angle of parallelogram ABCD be a and b as previous.
Let a and b be the lengths of the shorter and longer sides of the parallelogram
as previous. Constructing the squares on the sides of parallelogram so that the
squares lie on the inside of the sides of the parallelogram as shown in the
above diagram. Let EFGH be the midpoints of the squares.
Consider DGEH.
Length HA =
, GA = , <HAG = 90 - a, by similar reasoning used previously.
Consider DEDH.
Length HD = 
, DE = , <EDH = b - 90 = (180
- a) – 90
= 90 -a.
hence DGEH is congruent to DEDH
particularly GH = HE. Because the other two triangles highlighted are congruent
then quadrilateral EFGH has four congruent sides.
Now to show that EFGH has one right angle.
Consider <DEC = 900 by previous argument;
<HED < FEC by
corresponding parts of congruent triangles.
<DEC = <DEF + <FEC = 90 = <DEF + <HED =
<HEF
Thus quadrilateral FEHG is a square,
3. Extension: Comparing Areas
Consider the area of the final square EFGH formed in the
first investigation, and the final square (also called EFGH) in the second
investigation. Subtracting the area of the second square from the area of the
first square should give twice the area of the parallelogram.
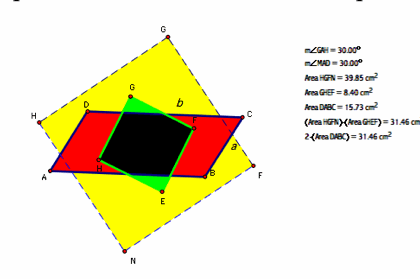
The GSP construction above verifies that for the area of
the first Square (yellow) subtract the area of the second square (green) is
twice the area of the parallelogram (red).
Problem
found at: